Spring 2024
Seminars take place online on Tuesdays at 3.00pm on Zoom via the link https://ucl.zoom.us/j/99614222402. Some of the seminars will be 'hybrid' (i.e. in person +zoom). If you require any more information on the Applied seminars please contact Prof Jean-Marc Vanden-Broeck (e-mail: j.vanden-broeck AT ucl.ac.uk or tel: 020-7679-2835) or Prof Ilia Kamotski (e-mail: i.kamotski AT ucl.ac.uk or tel: 020-7679-3937).
Tuesday 16 January 2024 in Rockefeller Building, Rm 333
Speaker: Betti Hartmann (UCL)
Title: scalar field stars and black holes with scalar hair
Abstract:
With direct gravitational wave detections as well as the first picture of the shadow of a black hole, there exists now complementary observational evidence that black holes - a priori a mathematical prediction of the best theory of gravity that we have to this day, General Relativity - do, indeed, exist in the universe. These recent observations seem to suggest that the corresponding black holes can be extremely well matched to the Kerr solution, a vacuum solution of the Einstein equation that describes a black hole determined only by its mass and angular momentum. Observable black holes hence seem to be very simple objects that carry no additional structure (“hair”). However, models appearing in theories that try to explain e.g. the nature of dark energy or the inflationary epoch in the primordial universe as well as recent studies within the gauge/gravity duality contain black hole solutions that often carry hair. Moreover, stars made out of scalar fields - so-called “boson stars” - provide viable alternatives to supermassive black holes.
In this talk, I will discuss boson stars and black holes that carry non-trivial scalar hair. I will motivate the models discussed and explain their applications.
Tuesday 23 January 2024 on Zoom
Speaker: Magda Carr (Newcastle University)
Title: Experimental and numerical modelling of internal solitary waves
Abstract:
Internal solitary waves (ISWs) are finite amplitude waves of permanent form that travel along density interfaces in stably stratified fluids. They owe their existence to an exact balance between non-linear wave steepening effects and linear wave dispersion. They are common in all stratified flows especially coastal seas, straits, fjords and the atmospheric boundary layer. In the ocean, they can attain amplitudes of up to 250m and speeds as large as 2 m/s. They are thought to be a source of mixing, and are important in resuspension of sedimentary materials, and mixing processes in the benthic boundary layer. They are subsequently of interest from both an environmental and offshore engineering point of view. In this presentation, experimental and numerical techniques will be described for modelling ISWs. Experiments will focus on stratified, buoyancy-driven flow, in a wave flume with visualization via particle image velocimetry (PIV). Numerical techniques will describe methods for solving Euler’s equation (via contour-advection methods) and the Navier-Stokes equation (via a spectral parallel incompressible solver). A summary of work by the speaker will be given on areas including (i) stability of ISWs, (ii) shoaling of ISWs and if time (iii) interaction of ISWs with floating bodies.
Tuesday 30 January 2024 in Rockefeller Building, Rm 333 & Zoom
Speaker: Ruoyu Wang (UCL)
Title: propagation and dissipation of acoustic and water waves on manifolds
Abstract:
In this talk, we discuss the wave semigroup with an unbounded damping. In such a setting, there are surprising examples where the linear damped waves would go into finite-time extinction. We will then find an optimal condition explicitly on the unboundedness to guarantee that the finite-time extinction cannot happen. We will also develop powerful yet flexible control-theoretic tools to establish novel polynomial stability and energy decay results for a variety of damped wave-like systems, including the linearised gravity water waves, Euler—Bernoulli beams, and Kelvin—Voigt damping.
Tuesday 6 February 2024 in Rockefeller Building, Rm 333 & Zoom
Speaker: Michael Gomez (King's College London)
Title: effective elasticity and dynamics of helical filaments under distributed loads
Abstract:
Slender elastic filaments with intrinsic helical geometry are encountered in a wide range of physical and biological settings, ranging from coil springs in engineering to bacteria flagellar filaments. The equilibrium configurations of helical filaments under a variety of loading types have been well studied in the framework of the Kirchhoff rod equations. These equations are geometrically nonlinear and so can account for large, global displacements of the rod, while maintaining a mechanically-linear (i.e., Hookean) constitutive law. This geometric nonlinearity also makes a mathematical analysis of the rod equations extremely difficult, so that the dynamic behaviour of helical rods under external loading is still generally poorly understood.
An important class of simplified models consists of ‘effective-column’ theories. These model the helical filament as a naturally-straight beam (aligned with the helix axis) for which the extensional and torsional deformations are coupled. Such theories have long been used in engineering to describe the free vibrations of helical coil springs, though their validity remains unclear, particularly when distributed forces and moments are present. In this talk, we show how such an effective theory can be derived systematically from the Kirchhoff rod equations using the method of multiple scales. Importantly, our analysis is asymptotically exact in the limit of a ‘highly-coiled’ filament (i.e., when the helical wavelength is much smaller than the characteristic lengthscale of deformation), and is able to account for large, unsteady displacements. We then illustrate our theory with two loading scenarios: (i) a heavy helical rod deforming under its own weight, and (ii) axial rotation (twirling) in viscous fluid, which may be considered as a simple model for a bacteria flagellar filament. More broadly, our analysis provides a framework to develop reduced models of helical rods in a wide variety of physical and biological settings.
Tuesday 14 February 2024 - No Seminar - Reading Week
Tuesday 20 February 2024 on Zoom
Speaker: Scott McCue (Queensland University of Technology, Brisbane)
Title: reinterpreting burgers' equation in the complex plane
Abstract:
Many of you will know the viscous Burgers’ equation, a very well-studied parabolic pde which sets up a competition between nonlinear advection that tends to steepen the solution profile and linear diffusion that tends to smooth it out. This pde is a simple toy model for the Navier-Stokes equation in 1D. A simpler version is the inviscid Burgers’ equation, which is a first-order nonlinear pde that can be solved exactly by an undergraduate using the method of characteristics. For this version of Burgers’ equation, there is no diffusion and so nonlinear advection drives the solution to continue to steepen until the derivative blows up somewhere in finite time. We shall revisit these models, but instead of restricting ourselves to the real line, we shall continue the solution out to the complex plane. In this way, we observe directly the culprit for the finite-time blow-up in the inviscid Burgers’ equation, which is a branch point that moves towards the real axis and touches it at the blow-up time. In the viscous Burgers case, the singularity structure in the complex plane is much more complicated, but with some investigative tools we can track the motion of complex-plane singularities and show why blow-up does not occur.
Tuesday 27 February 2024 on Zoom at 4pm-5pm
Speaker: Jon Wilkening (UC Berkeley, USA)
Title: the semi-analytic theory and computation of standing water waves
Abstract:
We generalize the semi-analytic standing wave framework of Schwartz and Whitney (1981) and Amick and Toland (1987) to the case of gravity-capillary waves or water waves of finite depth. We implement the algorithms in arbitrary precision arithmetic (up to 900 digits) on a supercomputer after developing an alternative formulation that employs 2D FFTs to rapidly evaluate the convolution-type lattice sums that arise. Sudden changes in growth rate in the expansion coefficients are found to correspond to (imperfect) bifurcations in the solution curves observed when standing waves are computed using a shooting method and numerical continuation. A direct connection between small divisors in the recursive algorithm and imperfect bifurcations in the solution curves is observed, where the small divisor excites higher-frequency parasitic standing waves that oscillate on top of the main wave. For certain values of surface tension, we prove that the small divisors are bounded away from zero. In the infinite depth case with zero surface tension, the algorithm appears to yield a convergent series. We compute its radius of convergence by polynomial extrapolation from the first 641 terms. For the unit-depth problem with zero surface tension, a 109th order Padé approximation maintains 25–30 digits of accuracy on both sides of the first imperfect bifurcation encountered. This suggests that even if the Stokes expansion for this case is divergent, there may be a closely related convergent sequence of rational approximations. This is joint work with Ahmad Abassi.
Tuesday 5 March 2024 in Rockefeller Building, Rm 333 & Zoom
Speaker: Radu Cimpeanu (University of Warwick)
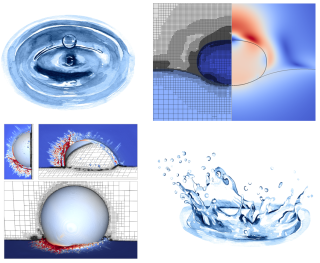
Title: from bouncing to making a splash: computational modelling of impact across scales
Abstract:
The canonical framework of drop impact provides excellent opportunities to co-develop experimental, analytical and computational techniques in a rich multi-scale context. The talk will represent a journey across parameter space, as we address beautiful phenomena such as bouncing, coalescence and splashing, with a particular focus on scientific computing aspects and associated numerical methods.
To begin with, we consider millimetric drops impacting a deep bath of the same fluid. Experimental measurements of the droplet trajectory are compared directly to the predictions of a quasi-potential model, as well as fully resolved unsteady Navier-Stokes direct numerical simulations (DNS). Both theoretical techniques resolve the time-dependent bath interface shape, droplet trajectory, and droplet deformation. In the quasi-potential model, the droplet and bath shape are decomposed using orthogonal function decompositions leading to a set of coupled damped linear oscillator equations solved using an implicit numerical method. The underdamped dynamics of the drop are directly coupled to the response of the bath through a single-point kinematic match condition, which we demonstrate to be an effective and efficient technique. The hybrid methodology has allowed us to unify and resolve interesting outstanding questions on the rebound dynamics of the multi-fluid system (Alventosa, Cimpeanu and Harris, JFM 957, 2023).
We then shift gears towards the much more violent regime of high-speed impact resulting in splashing, where a combination of matched asymptotic expansions grounded in Wagner theory and DNS allow us to produce theoretical predictions for the location and velocity of the ejected liquid jet, as well as its thickness (Cimpeanu and Moore, JFM 856, 2019). While the early-time analytical methodology neglects effects such as surface tension or viscosity (focusing on inertia instead), generalisations of the technique (Moore et al., JFM 882, 2020) and 3D extensions (ongoing work) will also be presented and validated against an associated computational framework. Should time allow it, recent results on coalescence and splashing for three-fluid setups (Fudge et al., PRE 104, 2021 and JCIS 641, 2023) will also be discussed.
Tuesday 12 March 2024 in Rockefeller Building, Rm 333 & Zoom
Speaker: Gavin Esler (UCL)
Title: A simple stochastic model of the quasi-biennial oscillation
Abstract:
The equatorial winds in the Earth's stratosphere are observed to oscillate from easterly to westerly with a period of around 28 months: a phenomenon known as the quasi-biennial oscillation (QBO). Remarkably, the physical mechanism for this oscillation has little to do with any astronomical forcing, but is instead a result of momentum transport by waves which are generated in the troposphere, propagate upwards, and then dissipate in the stratosphere. A simple mathematical model of the QBO, consisting of a single integro-differential equation, due to Holton, Lindzen and Plumb (HLP) is reviewed. Here a new ingredient is added to the HLP model - the wave source terms are made stochastic - reflecting the fact that the actual wave sources driving the QBO are highly intermittent. Our analysis shows that, for a wide range of stochastic processes, the effect of the random source terms is parameterised by a single `intermittency parameter'. The implications of the result for practical modelling of the QBO are discussed.
Tuesday 19 March 2024 in Rockefeller Building, Rm 333 & Zoom
Speaker: Ferran Brosa Planella (Warwick)
Title: Asymptotic methods for lithium-ion battery models
Abstract:
Lithium-ion batteries have become ubiquitous over the past decade, and they are called to play even a more important role with the electrification of vehicles. In order to design better and safer batteries and to manage them more efficiently, we need models than can predict the battery behaviour accurately and fast. However, in many cases these models are still posed in an ad hoc way, which makes them hard to extend and may lead to inconsistencies. In this talk we will see some examples on how asymptotic methods can be applied to obtain simple models that can be used in battery control and parameterisation.
 Close
Close

