Close
A rose by any other name
20 May 2013
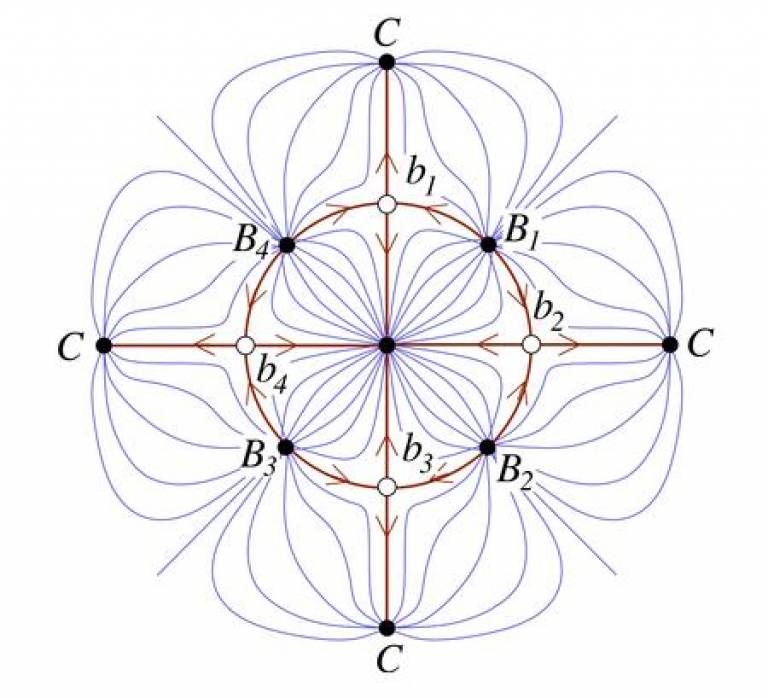
The picture represents a "finite energy foliation" of the 3-dimensional manifold S1 x S2 with its standard contact structure. Finite energy foliations are ways of filling 3-dimensional space with smooth surfaces that are solutions to a differential equation arising in string theory.
Such pictures can help mathematicians gain insight into problems in dynamical systems and in topology - for example, an important strand in research by Chris Wendl (UCL Mathematics) has been using finite energy foliations to try and answer the question, "how many different 4-dimensional shapes could this 3-dimensional shape be the boundary of?
One can use this particular picture to deduce that the answer is exactly one.
Credit: Chris Wendl (UCL Mathematics)
Copyright
- This image can be reproduced freely providing the source is credited.
Links
Tools
Open Days
The Faculty participates in a number of open days throughout the academic year, including the UCL Undergraduate Open Days and the UCL Graduate Open Day.
Friends of Out@UCL
Professor Ivan Parkin - Dean, UCL Faculty of Mathematical and Physical Sciences
"I fully support the aims of the Friends of Out@UCL campaign. I have personal experience of the need for such a campaign and the difficulties that the LGBTQ+ community face." Read more...
Snapshots from Space History
Online exhibition of historic space photos from the faculty's planetary science archives.