OLD! - For current see BIOSCIENCES COURSE SITE
 BIOL2007 - EVOLUTION OF GENETIC DIVERSITY

SO FAR WE HAVE:

a) Argued that the best definition of evolution is change in gene frequencies.
b) Shown how selection can lead to fixation, or replacement of an old allele by a new allele. IN THE NEXT LECTURE:
Kevin will discuss how mutation provides new raw material for evolution, in the form of new alleles.
HOWEVER: Do genes always evolve until they become fixed (invariant in a population), or become immediately lost because they are disfavoured? If so, most of the time, populations would be invariant and no change would occur.  However, in nature things are very different ...

IN FACT, THERE IS PLENTY OF GENETIC VARIATION IN NATURAL POPULATIONS! e.g. snail shell colour/banding, human eye/hair colour, protein variation in just about everything, DNA variation in absolutely everything Understanding the evolution of this genetic diversity Is a major goal of evolutionary genetics.

Possible explanations:

1) Selection on its own may directly explain diversity of alleles.  Here there is direct selection for polymorphism and diversity.

a) heterozygous advantage - selection for heterozygotes
b) diversifying frequency-dependent selection - selection for rare forms when their frequencies are low; ............OR:
2) Different forces may result in an equilibrium (i.e. a balance between forces leading to a zero rate of evolution): a) Mutation/selection balance - disadvantageous mutations are not eliminated immediately, especially if recessive. Mutation adds variants, and selection takes them away. A balance between input and output results.
b) Drift/mutation balance - some mutants will have little selection and might drift about neutrally; drift tends to fix mutants, mutation to bring them in.  This is the neutral theory of evolution. Here, the input of neutral mutations is balanced by genetic drift, which tends to remove mutations, or more rarely such mutations may increase to fix in the population.  Because drift can be slow in large populations relative to mutation,  polymorphisms may frequently result (see later in the course).
c) Migration/selection balance - disadvantageous genes can often be maintained in a population by migration from populations where the gene is favoured. Spatially varying selection is common, for instance in the peppered moth (see later in course). HERE WE WILL DEAL ONLY WITH:
(1a) heterozygote advantage
[also (2a) mutation-selection balance If we get around to it!!]

HETEROZYGOUS ADVANTAGE

It is easy to see that heterozygous advantage at a single locus with two alleles will lead to polymorphism:

AA           Aa           aa
-------> !!FITTER!! <-------
Why?  If you start with a pure population of AA, and add a few Aa heterozygotes so that pA is a little less than 1, Aa will do better than AA and a will therefore increase. The population that is fixed for A is at what is called an unstable equilibrium.  Similarly, if you start with aa common, rare Aa heterozygotes will do better, so A will this time increase.  A population with  pA=0 is also at an unstable equilibrium.  Somewhere in the middle between the unstable equilibria p=0 and p=1, there will be a stable equilibrium.

BUT we can go further than this simple verbal argument, and I am sure you will enjoy the maths, with me!

[In fact, almost the most interesting parts of evolution are still mathematically intractable.  For instance, it is only possible to obtain solutions for evolution of 2-3 genes, compared to 1000s present in most genomes. So don't worry, the maths doesn't get to hard! However, even if we can't get "analytical" solutions (for instance, equations for equilibria), we can still usually make approximations (see Quantitative Characters), or obtain "numerical"solutions (via computer simulations)].



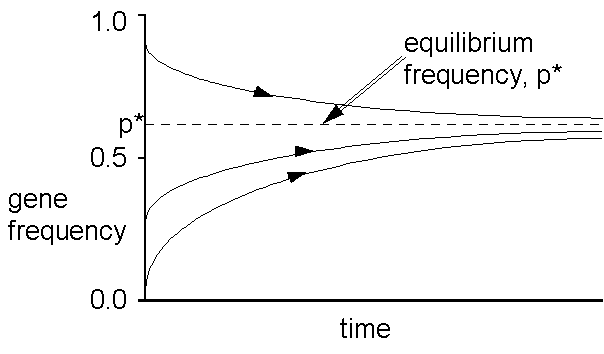
The above shows the time course of gene frequency change under heterozygous advantage for different starting conditions.  Notice the stable equilibrium p*? (the gene frequency at which no change results.)  You can play with this on your own using the simulation programs I have supplied.  We can calculate this equlibrium (i.e. when there is no further evolutionary change); using the symbols as before: 

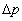= 0 when   p’    -    p   =   0
 i.e. (change in gene freq.) = 0  when  (new gene freq.)  - (old gene freq.) = 0 
Genotype         AA         Aa         aa
Frequency         p2        2pq         q2
Fitnesses       1-s          1         1-t
In the next generation, once again we count up the A and a alleles to get the frequency after selection,
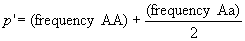, as before
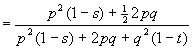  [ bottom line = the "mean fitness", as before]
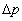= 0  when 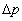= 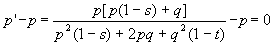at equilibrium

After a little manipulation, this nasty looking piece of work can be shown to be equivalent to a much simpler form:

pq(-sp + tq) = 0
Those of you who can remember back to your GCSE algebra (all of you, I hope!  If not, why not?), will immediately realize that the above expression is true if one of three conditions are met:
either    p = 0
or         q = 0
or         tq - sp = 0
The first two are fairly simple to understand.  The equation just says that if there are no a, q = 0 and the gene frequency remains fixed for A; if there are no A, p = 0, and the population remains fixed for a. This isn't really very surprising, since with no variation, there can be no evolution.  These fixations are therefore called the trivial equilibria, and as we have seen, they are unstable. Alternatively, the thing in brackets, (-sp+tq)  = 0, which is the same as saying tq = sp. This third equation therefore gives the internal or non-trivial equilibrium, p*, in which we are more interested because both A and a are present; the population would be polymorphic (frequency > 0) in the population.  Rearranging ...
tq* = sp*, so  t(1-p*) = sp*
therefore: t = sp* + tp*, so (s+t)p* = t
thus: .....
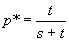: this is the polymorphic, or non-trivial equilibrium, which is stable
So, now if we know the selection pressures s,t, we can estimate the equilibrium frequency for heterozygous advantage; or we can work the equation the other way round.

You could try out simulating heterozygous advantage now in: natural selection simulations. The programmes show whether the fitnesses you enter produce directional selection or heterozygous advantage, and, if the latter, they plot the value of the equilibrium p* given above as a dotted line, so that you can see whether the gene frequencies converge towards it.

Now what do you think happens when the selection pressures s, and tare negative?  In other words, if the homozygote fitnesses are greater than the heterozygote fitness (this is called heterozygote disadvantage).

AA           Aa           aa
<------ !!LESS FIT!! ------>
Here when we introduce a few Aa heterozygotes to a pure population of AA, the AA do better, so the a alleles are lost. A population fixed for A is at a stable equilibrium; similarly, a population fixed for a is also stable.

Is there an internal equilibrium? Yes, our formula for p* shows it is again in the range 0 to 1; but this time, the equilibrium only remains if the population starts at exactly that frequency. Any slight perturbation, and it will diverge away from the equilibrium, and eventually go to fixation at p=0 or p=1. The equilibrium is here unstable, in which case the only stable equlibria are p=0 and p=1. This is quite an important case; because it also represents hybrid inviability; this is common when we cross different species, and is possibly an important cause of speciation.

You could try making s negative, and t positive or vice-versa. The same p* is still an equilibrium, but it isn’t a case of heterozygous advantage or disadvantage, it is a case like one we have dealt with already. Where is the "non-trivial" equilibrium now?  Calculate the relative fitnesses wAA, wAa, waa: what kind of selection is this? Is the "internal" equilibrium sensible? You could try out simulating these different fitnesses, heterozygous disadvantage, heterozygous advantage, using real values in natural selection simulations, if you wanted.

Now let’s try a real example, in humans: malaria resistance.


ESTIMATING SELECTION

The data is electrophoretic forms of haemoglobin: observations of beta-haemoglobin S (sickle-cell) and A (normal) genotypes in a malaria-infested region of West Africa (simplified from Cavalli Sforza & Bodmer 1971; available in Science Lbrary: View 2007 Teaching Collection by going to eUCLid; use Keyword, Basic Search, All Fields: 2007).  We have seen that one of the assumptions of Hardy-Weinberg equilibrium is that there is no selection.  If genotypic ratios deviate from Hardy-Weinberg, we may suspect selection provided we can rule out inbreeding, migration or other factors.  Here we use a 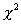 test to find whether there is evidence for deviation from Hardy-Weinberg:

Testing whether data conforms to Hardy-Weinberg ratios:

Table of genotype frequency calculations: 

Genotype                 AA           AS           SS           TOTAL
O(bserved number)      25,374       5,482          67          30,923
Expected fraction      0.8266      0.1651        0.0082         0.999*
E(xpected number)    (25,561.98) (5,106.03)     (254.98)      30,922.99*


Calculations of gene frequency and 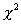 test:
Gene frequency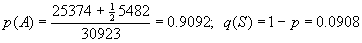 
Assuming Hardy-Weinberg, the expected genotype frequencies and expected numbers can now be calculated - see table above. (*Calculator notes)
Chi-square test: .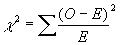


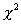= 1.4 + 27.7 + 138.6 = 167.7
Although there are 3 classes of genotypes, we lose degrees of freedom for any estimates we make from the data.  We lose 1 because we obtain the total from the data, and 1 because we estimate the gene frequency from the data, leaving 3-2=1 degree of freedom in this case.

Look for this value of 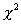 in your  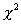tables, under 1 degree of freedom. You find that 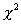=167.7 greatly exceeds the value for P=0.001, which is 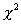=10.83. Therefore we can say that the probability of getting a 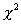 this big in a large number of trials if the "null hypothesis" (i.e. Hardy-Weinberg ratios) were true is much less than one in a thousand. In other words, there is very strong evidence for a deviation from Hardy-Weinberg -- we reject the null hypothesis.

We usually say all this more briefly: "there is a significant deviation from Hardy-Weinberg, P<0.001".

Estimating selection coefficients:

If we know that the population is not inbreeding, and we suspect selection against homozygotes; we can estimate the selection based on the data we have just analysed: 

Genotype                          AA           AS           SS
Work out fitnesses, O/E          0.99         1.07         0.26
now divide these through by by 1.07 to obtain .. 
Relative fitnesses                WAA              WAS              WSS
Values of fitnesses rel. to AS   0.92         1.00         0.24
Our selection model              1 - s         1           1 - t
Therefore the values of the selection coefficients are estimated as: s = 0.08, t = 0.76. (Check the answer?)

TAKE HOME POINTS FURTHER READING

FUTUYMA, DJ 1998. Evolutionary Biology.  Chapter 9 (pp. 231-247); Chapter 13 (pp. 376, 381-390).
Science Lbrary: View 2007 Teaching Collection by going to eUCLid; use Keyword, Basic Search, All Fields: 2007


Back to BIOL 2007 TIMETABLE